(Static -vrs- Dynamic Equations)
12B
~ The Study of Threes ~
http://threesology.org
Visitors as of 2/27/2021

Instead of using a bullet list format I chose to use an enumerated one to make a point about all lists being conjured up by us humans. They are all conserved ...Like the periodic table of 118 elements, the triplet code of DNA, axioms used in mathematics, and various natural laws as well as S.O.Ps being used in different industries. All of them reflect a conservation.
For example, whether we discuss three categories of learning styles: visual- auditory- tactile (Unlearning the learning styles) By Don Lincoln, Ph.D., August 27, 2020, or The Big Five Personality Traits By Kendra Cherry (Reviewed by David Susman, PhD), on February 20, 2021; one list after another list from one subject to another, are all expressions of a recurring "mathematically-linked" behavior of conservatism. If someone points them out just about everyone is likely to recognize such a conservation, but otherwise, the recurring conservation is being overlooked... and if someone is questioned about their usage of a particular conservation... they most likely will offer some plausible reason. In other words, the lists are short... again... just like the groups of axioms we see in mathematics. Yet this conservation is not being used as a variable for understanding the patterns recurring in human thinking... such as mathematics itself.
The absence of such a variable in our equations make them all static. We need a new brand of Calculus which includes the variable of the recurring conservation taking place in mathematics as an attempted survival response to an incrementally deteriorating environment. Even pure mathematics does not include such a variable.
We are packing more and more information into smaller criteria, like some compartmentalizations seen in prokaryotic DNA replication and tightly packaged genetic material in the linear chromosomes of a prokaryotic genome as well as the compression of DNA in the genome of bacteriophages. This is what we see in some types of mathematics equations. Attempting to create an increased density by overlapping assumed complexities. But this is an artificialized attempt at forestalling the inevitable whittling away at resources, as if creating complexity will somehow provide us with a barrier or buffer zone.
Attempting to dismiss the necessary discussions and worthwhile efforts to provide some remediation by saying that the incremental environmental deteriorations will take place over a long period of time and then associate human life span with some sort of geological event span of time is pure stupidity. We can not measure the effects of deterioration on biology by comparing it to geological events. Math equations are not reflecting the effects of deterioration which are reflective of human thinking processes. We do not have the necessary calculus to provide us with an accurate indication of how the environmental deteriorations are affecting mathematical reasoning as a bi-product of a biologically-based brain.
In the following selection let me first recount the foregoing types of intelligences by Howard Gardner's theory: (Multiple Intelligences) and then next to it put a mathematically-centered twist to them, though one could just as well use any subject and do the same to indicate generalized-specificities within a specifically-generalized genre (and though such an ending expression may be interpreted as being humorous by some readers, I am actually pointing out an inherent dichotomy which can be exercised):
|
|
In the foregoing table, some readers will not have any difficulty at all with recognizing the internalities of mathematical correspondences which occur along the separate types of labeled mathematical exercises.
In speaking to the development of a new model of calculation whose variables, enumeration, and symbols exist in a multitude of subjects with their respective languages, idioms, slang, and overall vernacular, it is of need to point out a very basic and generalized over-view of mathematics from its earlier beginnings as one might make an educated guesstimation of. For example: Before mathematics there was accounting. Before accounting there was simple counting.
It reminds me of the early processes under-which a cell emerged with its organelles... by "friendly" aggregation and/or predatory capture... intentional or serendipitously.
Before simple counting there was collation (simple or by way of a "hoarding instinct"). Before collation their was collecting. Before collecting there was observation. Yet despite the foregoing approximations, let me attempt some model of itemization of these items in order to provide an inferentially associated reference, along with some instances of when they may have arisen in time:
- Observation: (millions of years ago) of natural phenomena such as low and high tides, night and day, hot and cold, stars, birds, life/death, young/old, etc...
- Collecting: (millions of years ago) rocks, leaves, shells, feathers, etc...
- Collation: (millions of years ago) into piles, pairs, singularities referenced by good/bad, weak/strong, important/not important, etc...
- Simple Counting: began around 50,000 years ago. (word/symbol? reference) 1 → 1 and many/heap/much/more; 1, 2→ 1 and 2 and many/heap/much/more; ... start- start, etc...
- Early Accounting: rudimentary number collections tied to early counting: Hence... A- (type of) counting.
- Astrology/Zodiac: Number, constellation (human/animal/month) pairing...
- Meso-American calendars: Zodiac? (Aztec, Incan, Mayan) 3 noted types:
- 260-day calendar, a ritual calendar with no confirmed correlation to astronomical or agricultural cycles
- 365-day period approximating the tropical year, known sometimes as the "vague year".
- Long Count is found in the inscriptions of several Mesoamerican cultures, most famously those of the Maya civilization who developed it to its fullest extent during the Classic period (ca. 200–900 CE). The Long Count provided the ability to uniquely identify days over a much longer period of time, by combining a sequence of day-counts or cycles of increasing length, calculated or set from a particular date in the mythical past. Most commonly, five such higher-order cycles in a modified vigesimal (base-20) count were used. Mesoamerican calendars
- Aztec Zodiac: (14th century? → origin of civilization
- Incan Zodiac: 12th to 15th centuries (Inca Astronomy)
- Mayan Zodiac: 2600 BC? → origin of civilization
- (List of pre-Columbian cultures... though the list of Mesoamerica excludes the Incas: List of pre-Columbian cultures)
- Babylonian (Mesopotamian): [12 months] 2000 BC Babylonian astrology
- Chinese Astrology/Zodiac: 5th century BC The Chinese Zodiac
- Egyptian Dendera zodiac... 2500 BC?
- West Eurasian peoples: 3000 BC... (History of astrology)
- Geometry: approximately 3,000 BC in ancient Egypt. (occurring sometime later after simple star-gazing. (Astronomy said to have arisen around 4th century BC defined by representations of planetary system.) From Star gazing arose sacred numbers like seven (7-starred Big Dipper, 7-starred Pleiades)...
- Later Accounting: single-entry accounting (300 BC) followed by double-entry accounting (1400s)...
- Algebra: 1600 to 1900 AD
- Calculus: late 17th century by Isaac Newton and Gottfried Leibniz.
Simply put, we can see the development or mathematics from simple beginnings, that are not routinely brought up in many articles about the history of mathematics, though this article does try to be a bit more objective in its considerations because it asks the question of where to start one's research in the history of mathematics: Early Counting Systems.
Indeed, did animals (or even plants?) have a sense of quantity... that we might infer to be a rudimentary form of counting; long before humans did? Or did very early humans have a sense of number... of quantity, though such a behavior was not consciously acknowledged? Did competition for food, for mating, for one or another resource play a role in the development of quantity in the sense that when two or males began to butt heads, the idea of one (or two) too many became a standard concept that was later used as an unrecognized impetus for the development of quantification?
When we take stock of early counting methods, we come across stops and starts (like the one start and three stop codons found in genetics). For example, a person develops a symbol and word related to the quantity of one (1), and all other quantities more than one may or may not have received a symbol (such as our 2, 3, etc., and infinity symbol). Thus, we assume that early counting efforts exhibited a stop at "one", whatever word was used or however it may have been symbolically represented.
Next comes two with perhaps an associated symbol (such as perhaps one or more held-up fingers) along with a word (perhaps a grunt). Then the earlier counter stops at this point, which may or may not be associated with a concept for everything else such as heap, a lot, much, many, etc... In other words, they had a quantity for the value "one", but any symbol and/or word thereafter referred to all other quantities. Hence, we are confronted with another cognitive stop which may have persisted for several generations.
Next comes the concept of something beyond the "two" (which once served to address all quantities more than one), such as the notion of "three" with or without a corresponding symbol (other than with perhaps one or more held-up fingers) and word... which may or may not be associated with a word and/or symbol for everything that is more than three which also then represented another cognitive step. Whereas they had "one" and all quantities beyond one was some culturally equivalent "many" (or whatever their notion was), this was then followed by another concept which then became an enlarged sequence (of two or three values)... followed each time by some sort of cognitive limitation. At present, we of today use the word "infinity" to describe some notion of conceptualizing a larger sense of quantity.
Anyway, the point is that in the development of numbers, we shouldn't expect to see (archaeologically or anthropologically speaking), someone (some culture) having woken up one morning with the concept of numbers for 10, 100, 1000, etc., and their associated word/symbol references. Whereas some assumed genius may well have done so, their views were not recorded... only those use (mostly) by accountants or architects. Whereas there may have been many geniuses in the past, their views were either lost, never recorded or deliberately concealed... like some students whose ideas and efforts are stolen by some authority figure that becomes backed up by other authority figures in a given subject area. Needless to say, the concept and usage of counting took time to develop, particularly into a standard usage. And yet, though cultures the world over learned to count and then later develop mathematics which they may or may not have borrowed from other peoples; not every single practice developed an interest in "higher" math computations. In other words, not all cultures developed their versions of higher mathematical thinking expressed either with symbols and notions we are familiar with today, or their own variations which later became altered into some present day standard notation.
If we look at different interests which use numbers, and though the practice may have been around for centuries, the simple usage of numbers did not evolve into what we of today generally describe as a mathematics. Take for example the zodiac. It retains a simple number ordering system aligned with numerically designated months, birth dates, etc., but its practitioners did not gravitate towards producing a formula of mathematics comparable to algebra, trigonometry or calculus. In other words, the Zodiac retains a rudimentary form of counting and addition, though some basic geometry is attempted by charts of alignment. The same goes for business accounting. In short, we can find examples of using numbers in different types of practices, but they have not developed into a full blown exercise of mathematics, even though some partitioners of a given craft may well have a thinking process equivalent to some form of higher mathematics. They simply use another vocabulary and rely on a model of symbolism that has not had the advantage of being taught to millions of people in school systems... from which to use the efforts of these millions to shape their interest into a finer piece of sculpture out of their crude forms.
Indeed, we find that many inventions and activities do not use mathematics as a means of expression, but that they in fact express a presumed higher form of logic and consideration. Their subject has not had the advantage of funding and larger repositories of exercised effort to make improvements by the actions of multiple minds. If no one had taken an interest in mathematics, and public officials were not influenced by business practices to ensure everyone was grounded in some level of mathematical knowledge for the purpose of at least using money and simple bartering; Mathematics may well not have become what it is today. Likewise for all "higher" academic pursuits as well as other professions. If no one (after the Greeks) had taken an interest in the Olympics, it would not be what it is today. Likewise for theater, politics, warfare, and other sports. Needless to say, that if humanity followed the lead of the ancient Greeks in everything, we may not have chemistry today, except as some dead-ended philosophical exercise... much less biology, anthropology, etc... (If the ancient Greeks had not taken a scientific approach to medicine, what would be the state of medicine today?)
However, we have allowed mathematics to dominate the type of reasoning we have adopted in accordance with a preference for using mathematics as a measuring tool of truth and logic; the lack of using mathematics can create conditions which deny a person to get their ideas across to those who primarily accept mathematics as "THE" tool for expressing truth. Even though millions of people work out ideas by intuition and visualization by which useful creations are made, we have become so reliant on mathematics to define what is real, what is true, what is accurate. Millions of people are denied a full expression of exhibiting their freedoms, to the extent some people don't try to exercise their "Eureka!" sparks of realization because society teaches everyone from elementary school on that if they can't express themselves, their ideas, their dreams in a mathematical way, they are less worthy of some "higher" distinction which only mathematics can convey. Mathematics is a type of enslavement... and mathematicians... along with education systems, are the whip-wielding task masters. You are viewed as being incapable of grasping higher concepts in anything unless it is accompanied by an astute comprehension of mathematics. It is believed by some that only Mathematics can provide the supposed higher realms of visualization which human biology is capable of. Perhaps we need a system of higher education where an alternative to mathematical-types of visualizations are not permitted to be expressed by mathematical symbols and vocabulary.
Like the old requirement of being able to read, write and speak Latin, Mathematics has become the new Latin requirement for knowing a foreign language, though other subjects are viewed as being just as strange by other students, such as chemistry and philosophy... for instance. (However, unlike mathematics, the difference being that if you don't know one or more of the other subjects you are not necessarily barred from entering into other courses.) Mathematics is being used as a sort of (openly conveyed) secret handshake, secret password, or some other secret requirement before you are permitted to enter into a given subject area or a given subject area's "higher" classes. It is thought that if you can't perform some level of mathematics you will be unable to visualize the necessary ideas being conveyed in higher levels of some courses.
Let's take a look at some examples of different great thinkers who either found mathematics a struggle, even distasteful, or whose ideas were dismissed as presenting some irrelevancy because the inventor or theorist couldn't read, write nor speak the language of "Mathematish" (I used the suffix "ish" which is used to denote languages such as English and Spanish, instead of simply using the word Mathematics.): 6 Famous Scientists and Inventors Who Struggled With Math by Mark Mancini, Oct, 19, 2015
- Michael Faraday (1791-1867) In 1846, he boldly proposed that visible light is a form of electromagnetic radiation. But because he couldn't back up the idea with mathematics, his colleagues ignored it.
- Charlie Darwin (1809-1882) As a collegiate student, he loathed Mathematics. "The work was repugnant to me.”
- Alexander Graham Bell (1847-1922) According to biographer Robert V. Bruce, Bell "enjoyed the intellectual exercise" of this subject, but was "bored and hence careless in working out the final answer once he learned the method." His grades suffered accordingly. Bell's mathematical aptitude never improved.
- Thomas Edison (1847-1931) "I can always hire a mathematician," Edison once remarked, "[but] they can't hire me." In his own words, according to one Thomas Edison biography, the book left him with nothing but "a distaste for mathematics from which I never recovered."
- Jack Horner (1946-Present) Horner's success (in Paleontology,) must have shocked his childhood teachers. The Montana native did poorly in school, which he found "extremely difficult because my progress in reading, writing, and mathematics was excruciatingly slow."
- E.O. Wilson (1929-Present) ...2013's Letters to a Young Scientist—reveals a tumultuous personal history with math. The product of "relatively poor Southern schools," Wilson admits that he "didn't take algebra until my freshman year at the University of Alabama... I finally got around to calculus as a 32-year-old tenured professor at Harvard, where I sat uncomfortably in classes with undergraduate students only a bit more than half my age.
The Wright Brothers of airplane flight and Kittyhawk fame appear to have relied on data constructed by Otto Lilienthal of Germany and John Smeaton an English civil engineer. While history records the brothers using the data, it does not explicitly say how and in some reports, it is said that they thought lilienthal might be wrong. (The Wright Stuff: The Mathematics of the Wright Brothers by Robert N. McCullough. Other reports claim: "The truth of the matter is that it was not a problem of Lilienthal errors, rather it was a misinterpretation of his data that was the problem." Lilienthal Data not in Error by DR. Richard Stimson. Whatever the case may be, an examination of their pocket-sized notebooks exhibit simple graphs and tables of trial and error efforts and do not admit to the usage of any exhaustive "higher" mathematics, perhaps owing to the fact that neither of the brothers went beyond high school and did not show a proclivity towards learning math beyond rudimentary skills. No less, their partner in the enterprise... their sister Kathrine wright was a school teacher, but with respect to math: Katharine excelled in most of her courses of study, with the exception of math. She met with a tutor three times a week and that tutor, Henry Joseph Haskell, would later become her husband.
When we attempt to discover the Mathematics used by Leonard Da Vinci, we encounter present day interpretations of his works in relation to our mathematical representations of his efforts. In other words, we do not see math equations, but illustrations of perspective, space, intuition, proportion and geometrical shapes. Let us be honest and don't try to embellish his works by describing them in modern terms just because we have some special affinity for mathematics. He didn't use mathematics as we today ascribe to it. People need to stop romanticizing that he (and other famous people) used mathematics in the way we of the present do. You don't hear of every artist today being described as a mathematician who use similar devices for constructing their works. Interpreting everything Leonardo did in a mathematical framework is just as silly as those who believe they uncovered some hidden message in the dimensions of the pyramids, or obelisks, or hieroglyphics. However, what it amounts to is a confession that what we think is a fundamental truth of mathematical thinking... of mathematical logic; does not need so-called "proper" math expressions called (conventionalized) equations. It can be expressed alternatively, except that present day education is ramming some idealized "proper" mathematics as the only form that every child must have, without exception.
Let us take a brief look at the development of mathematics during Leonardo's time (15 April 1452 – 2 May 1519):
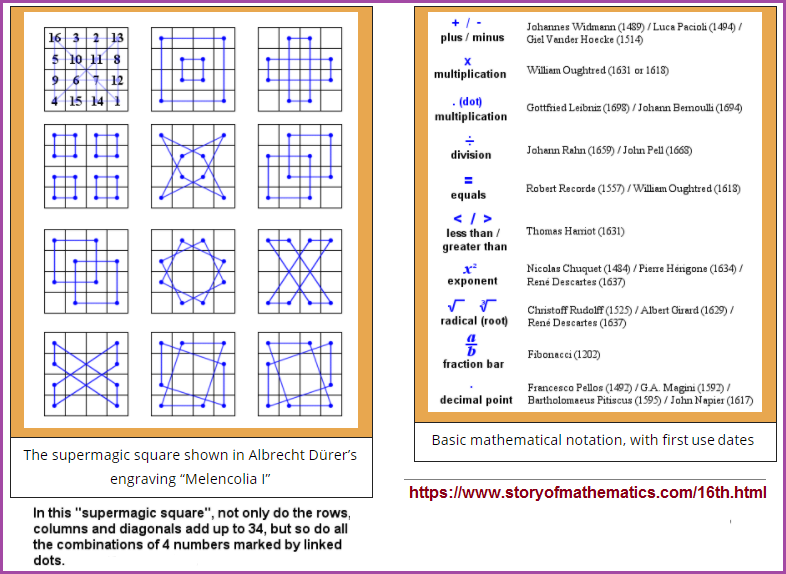
The Supermagic Square
It is a tribute to the respect in which mathematics was held in Renaissance Europe that the famed German artist Albrecht Dürer included an order-4 magic square in his engraving "Melencolia I". In fact, it is a so-called "super magic square" with many more lines of addition symmetry than a regular 4 x 4 magic square (see image above). The year of the work, 1514, is shown in the two bottom central squares.
An important figure in the late 15th and early 16th Centuries is an Italian Franciscan friar called Luca Pacioli, who published a book on arithmetic, geometry and book-keeping at the end of the 15th Century which became quite popular for the mathematical puzzles it contained. It also introduced symbols for plus and minus for the first time in a printed book (although this is also sometimes attributed to Giel Vander Hoecke, Johannes Widmann and others), symbols that were to become standard notation. Pacioli also investigated the Golden Ratio of 1 : 1.618... (see the section on Fibonacci) in his 1509 book The Divine Proportion, concluding that the number was a message from God and a source of secret knowledge about the inner beauty of things.
During the 16th and early 17th Century, the equals, multiplication, division, radical (root), decimal and inequality symbols were gradually introduced and standardized. The use of decimal fractions and decimal arithmetic is usually attributed to the Flemish mathematician Simon Stevin in the late 16th Century, although the decimal point notation was not popularized until early in the 17th Century. Stevin was ahead of his time in enjoining that all types of numbers, whether fractions, negatives, real numbers or surds (such as √2) should be treated equally as numbers in their own right.
In the Renaissance Italy of the early 16th Century, Bologna University in particular was famed for its intense public mathematics competitions. It was in just such a competition that the unlikely figure of the young, self-taught Niccolö Fontana Tartaglia revealed to the world the formula for solving first one type, and later all types, of cubic equations (equations with terms including x3), an achievement hitherto considered impossible and which had stumped the best mathematicians of China, India and the Islamic world.
The Story of MathematicsSince mathematics is a made-up language, like runes, ideographics, Las Calle wall paintings, hieroglyphics, ancient Greek, Latin, Aramaic as well as the languages-laden vocabularies of all subjects (developmental biology, religion (the many sects of Islam, Bahai' faith, Christianity, Buddhism, Judaism, Mormonism, Shintoism, Jainism, Hinduism, Quakerism, etc...), evolution, politics, physics, sports, theater, warfare, etc..)— to include all cultures, archeological and paleo-anthropological artifacts, Astrology, Astronomy, Free-Masonry and Knights Templar symbology, etc.; let us also note that many of our subjects either grew up in an age where the existence of cult thinking and participation was a regularity defined by a small selection of individuals, or the traditions of cult-mindedness greatly influenced later ideas and ideals to re-enact internalized secrets and secrecy... which today may be defined by larger memberships due to an overall increased global population, and an exposure of secret activities which may be called entrance fees, learning fees and rituals with extensive memorizations, secret password or handshakes replaced by employee badges, dress codes, and multiple other language codes generalized into the two-part phrase of "Walk the walk and Talk the talk" (of the initiates in a given enterprise). In some cases such as street gangs and criminal activities, a person is expected to join for good, for life, or forever, or be confronted by a death sentence, severe ostracism, or other occurrences amounting to a life-long penance similar to the mental mood of those subjected to long-term stretches of incarceration which become minimized by inmates by having time equated with small denominations of money. For example, a five year sentence may be viewed as a "nickel". A ten-year period is called a "Dime". Twenty five years is a quarter and so forth. Minimization is an expression of conservation thinking. Many prisoners routinely minimize their crimes, just like business people and the illogicality of legislation pursued by some politicians.
Mathematics, like religion, has been a successful cult. It has even managed to become a socially established requirement for all people to become proficient in at least in terms of simple or basic arithmetic. Religion has achieved a similar imposition on the public by establishing the idea of a supreme god, and that religion is the owner of such an idea as well as claiming itself to be the master of spiritual truth and overall human morality. But there are other cult-minded institutions such as the dominance of the word "Democracy" and "Capitalism". All politics and economic perspectives are cults. Albeit cults with millions of followers, but cults nonetheless... just like sports and gaming institutions.
With respect to Mathematics being viewed as a cult having its own practiced (living) speaking language, the requirement to learn its written language and language-related symbology, is time and again found to be difficult for most people to learn the abstract language configurations, much less acquire a familiarity to write the language as required by a given 'sect' (such as the mathematics used in physics, population genetics, fluid mechanics, geometry, etc...). So, is this a problem with the larger public, or the smaller languages of mathematics? In other words, is mathematics at fault because it requires a type of memorization and twists of thought processing out-of-step with the conventions of what might be called a Standard Cognitive Model of thinking? Is present Mathematics just a modernized form of ancient Latin which will eventually fall by the wayside as well... though some other model of mathematical language may nonetheless be developed?
It is not uncommon to find someone who thinks that Mathematicians are like artists constructing artistic expressions or musical renditions in deliberately conjured-up contraptions called equations and musical scores in methods and manners suited for a specific audience with a given taste. In other words, Mathematics is looked like a speciality of appreciation that is being forced on the whole of the public to swallow like U.S. children of the past were forced by parents to drink regular spoonfuls of castor oil as if it were a universal preventative measure for multiple illnesses. Many, if not most math equations are being viewed as Picasso-like abstractions where a select few of interested people have a dominant sway on the overall interpretation of its social usefulness... though many a Mathematician (for example, G.W. Hardy Of Ramanujan fame), hoped their "Pure" mathematical expressions are never found to have a utilitary application, because they believe they are engaging in a superior form of math as if it were like a transcendent being that exceeds all realms of spiritual and philosophical species of body, mind, and soul.
Hence, to suggest we alter the speaking and written as well as feeling, thinking and intuitive characteristics of Mathematics by introducing the concept of a Dynamic (or dynamical) mathematics which a circle/sphere or a one/two-sided triangle, 3-sided (plus base) trihedron, or 4-sided (plus base) tetrahedron may be used to generalize an illustration thereof; may give the impression to some that such a task is the engagement in mere semantics than actually providing a means by which we are enabled to step into a new territory of mathematics expression suggested by the notion of a dynamic versus a static equation, (without intending to be interpreted as a combative stance)... the latter being a reference to the present currency of linear equations being used as two-dimensional configurations requiring an individual to supply visualized animations within the linear language vernacular (tongue, jargon, etc...).
And yet, if such a language as the linear is counter-intuitive, presenting multiple obstacles to many people as evidence by the larger public's aversion to learning the language because the memory techniques for embracing the language are themselves a difficulty of the language requirement, they are said not to be able to "see", to appreciate, to fully realize the supposed greater truth (like some who claim that a [particularlized] "spiritual experience" is a requirement for someone being able to fully grasp their beliefs); because they are not expressing it in the required mathematical language nor thinking in the language. Hence, the refrain that "something is lost" in translation when the language is poorly understood and instead of being the urban legend compilation which it is; mathematics is held up as some sort of definitive declaration which asserts the self-initiated validations of Mathematicians of a self-molded embodiment of presumed uniqueness that becomes an accepted standard traditionalized by a general public mood of an indentured obeisance to this view.
Being able to grasp (if not write and speak of) reality in the way mathematicians do, is more easily absorbed if one is subjected to a culture in which mathematics is the standard form of everyday communication. It is its own type of sanity... or if you prefer: it's own flavor of insanity. If you have ever been to a place of learning where everyone embraces the given language and beliefs of a particular subject, fanaticism is a common personality theme. For example, to attend a college where the dominant orientation is to a given religious belief, you might well find yourself in what you would otherwise describe as a psychiatric ward, where all perceptions are rationalized in terms of the dominant belief and conveyed in the dominant language used to convey the many different day-to-day life-skill narratives, songs, relationship communications, personal goals, self-denials, self-recriminations, group support networks, etc... If you don't believe in the religion, or your mind is not configured to play out a role in engaging yourself in what can be described as playing with a Rubick's cube, you may well find yourself thinking everyone around you is insane... or that you are. Yep, Mathematics is its own insanity, despite its usefulness because humanity has not thought up something better.
Beyond simple arithmetic... to include fractions, perhaps some decimals and basic graphs and geometry; is how most people live out their lives. While some may want to know more mathematics and envy those who have a penchant for mathematics, the truth of the matter is that they carry on with what basic math skills they have and turn to the use of something else... such as words, or puzzle making, or cooking, or gardening or rebuilding a vehicle from the ground up, or becoming a self- or publicly noted- expert in one thing or another that does not involve math. For example, the work of plumbers, electricians police officers, politicians, doctors, dentists, mechanics, delivery drivers, garbage collectors, fruit harvesters, etc., are not noted for their math skills, even though they may well have developed a personalized form of higher thinking... or so they assume, but do not "show their work" in a way which will enable others to point out flaws. Rough carpenters have finish carpenters to cover up their crude building efforts which exhibit a fluctuating generality and not an exactness.
Yet, again, each one of the non-mathematical equation professionals may consider something they do is equivalent to higher... if not "pure" mathematics, albeit within the scope and with those implements characteristic of a given exercise; be it mental, emotional or physical... mix and match as you will. Indeed, do we claim that math is an expressed intelligence used by some as a tool, whereby math is discovered instead of being created? Or is it that someone creates it so that another may discover it at some other time? In this sense it would be that the development of a new mathematics... as a new or different type of human conceptualization, emerges on the scene of human ability that may become mentioned to another who likewise is near enough the evolutionary change in mental development, that they to are led along the same or similar path and make note of it... and it is the note in the hands of one or more that gets tossed socially about "under the radar" of most conversations
Is Mathematics Invented or Discovered?
Date of Origination: 26 February 2021... 6:59 AM
Initial Posting: July 21st, 2021... 2:12 PM